When pipes are installed by HDD, they often experience high tension loads, severe bending, and external fluid pressures. Often these installation loads are more severe than the design service loads. When selecting the appropriate pipe materials for an HDD installation, the designer must consider the pipe properties as well as the borehole profile. These two factors should be considered together in order to choose the best material and profile so that the pipeline can be installed and operated without risk of damage. To ensure that the material and bore-hole profile are suitable for the proposed application, the installation, operational, and combined loads and stresses are analyzed.
Calculation Overview
Pipe Weight in Air:
𝑃𝑖𝑝𝑒𝑤𝑒𝑖𝑔ℎ𝑡 – Weight of the pipe (lbs/ft)
Pipe_{weight}=10.68(D-t)t𝑃𝑖𝑝𝑒𝑤𝑒𝑖𝑔ℎ𝑡 – Weight of the pipe (lbs/ft)
D – Pipe Outside Diameter (inch)
t – Pipe Wall Thickness (inch)
Pipe Exterior Volume:
Pipe_{ext.vol}=\left(\frac{D}{24}\right)^2\pi
Pipe_{ext.vol}=\left(\frac{D}{24}\right)^2\piPipe Interior Volume:
Pipe_{interior.vol}=\left(\frac{D-2t}{24}\right)^2\pi
Pipe_{interior.vol}=\left(\frac{D-2t}{24}\right)^2\piWeight of Water in pipe:
(to be calculated only if the pipe is filled with water)
Water_{{p \cdot weight}} = Pipe_{{interior \cdot vol}} \times W_{\text{weight}}
Water_{{p \cdot weight}} = Pipe_{{interior \cdot vol}} \times W_{\text{weight}}Wweight – Weight of water (lb/ft3)
Displaced Mud Weight:
Displacemud_{\text{weight}} = Pipe_{{ext \cdot vol}} \times mud_{wt}
Displacemud_{\text{weight}} = Pipe_{{ext \cdot vol}} \times mud_{wt}mudwt = Weight of mud (lb/gal)
Effective Weight of Pipe:
W_S = Pipe_{\text{weight}} + Water_{{p\cdot weight}} – Displacemud_{{weigh
W_S = Pipe_{\text{weight}} + Water_{{p\cdot weight}} - Displacemud_{{weight}}Straight section A-B
Friction from Soil:
\text{fric}2 = W_S L_1 \cos \theta{S1} \mu_{\text{Soil}}
\text{fric}_2 = W_S L_1 \cos \theta_{S1} \mu_{\text{Soil}}𝐿1 – Length of the straight section 1
𝜃𝑆1 – Angle in degrees from horizontal for straight section 1
𝜇𝑆𝑜𝑖𝑙 – Average coefficient of friction between pipe and soil. The recommended value is between .21-.3 (Maidla)
Drag Forces from Mud:
\text{Drag}2 = \pi D L_1 \mu{\text{mud}}
\text{Drag}_2 = \pi D L_1 \mu_{\text{mud}}μmud – Fluid drag coefficient for steel tube pulled through bentonite mud
Tension on Section:
\Delta T_2 = | \text{fric}2 | + \text{Drag}_2 – W_s L_1 \sin \theta{s1}
\Delta T_2 = | \text{fric}_2 | + \text{Drag}_2 - W_s L_1 \sin \theta_{s1}Cumulative Pull Load: T_2=\Delta T_2+T_1
T_2=\Delta T_2+T_1
T1 – Pull back as the pipe enters the drill hole (lbf)
Curved Section B-C
h3 = R_1 \left( 1 – \cos\left(\frac{\theta_{C1}}{2}\right) \right) \~\ I_3 = \pi (D – t)^3 \frac{t}{8} \ j_3 = \left( E\frac{ I_3}{T_{\text{avgassumed3}}} \right)^{\frac{1}{2}} \~\ U3 = \frac{L_{\text{arc1}}}{J_3} \~\ X3 = 3 \frac{L_{\text{arc1}}}{12} – \left( \frac{j_3}{2} \right) \tanh\left( \frac{U_3}{2} \right) \~\ Y3 = 18 \left( \frac{L_{\text{arc1}}}{12} \right)^2 – j_3^2 \left( 1 – \frac{1}{\cosh\left(\frac{U_3}{2}\right)} \right) \~\ N_3 = \frac{T_{\text{avgassumed3}} h_3 – W_s \cos\left(\frac{\theta_{C1}}{2}\right) Y_3}{X_3}
h3 = R_1 \left( 1 - \cos\left(\frac{\theta_{C1}}{2}\right) \right) \\~\\ I_3 = \pi (D - t)^3 \frac{t}{8} \\ j_3 = \left( E\frac{ I_3}{T_{\text{avgassumed3}}} \right)^{\frac{1}{2}} \\~\\ U3 = \frac{L_{\text{arc1}}}{J_3} \\~\\ X3 = 3 \frac{L_{\text{arc1}}}{12} - \left( \frac{j_3}{2} \right) \tanh\left( \frac{U_3}{2} \right) \\~\\ Y3 = 18 \left( \frac{L_{\text{arc1}}}{12} \right)^2 - j_3^2 \left( 1 - \frac{1}{\cosh\left(\frac{U_3}{2}\right)} \right) \\~\\ N_3 = \frac{T_{\text{avgassumed3}} h_3 - W_s \cos\left(\frac{\theta_{C1}}{2}\right) Y_3}{X_3}
(based on Roark’s solution for elastic beam deflection)
θC1 – Angle in degrees from horizontal for curved section 1
R1 – Radius of curvature of curve section 1 (ft)
Larc1 – Length of curved section 1(ft)
E – Young’s Modulus (psi)
Friction from Soil:
fric=|N_3\mu_{Soil}|
\text{fric}=|N_3\mu_{Soil}|Drag Forces from Mud:
Drag_3=\pi DL_{arc1}\mu_{mud}
\text{Drag}_3 = \pi D L_{\text{arc}1} \mu_{\text{mud}}
Tension on Section:
\Delta T_3 = 2|\text{fric}3| + \text{Drag}_3 – W_s L{\text{arc}1} \sin\left(\frac{\theta_{C1}}{2}\right)
\Delta T_3 = 2|\text{fric}_3| + \text{Drag}_3 - W_s L_{\text{arc}1} \sin\left(\frac{\theta_{C1}}{2}\right)
Cumulative Pull Load: T_3=\Delta T_3+T_2
T_3=\Delta T_3+T_2
Straight Section C-D
Friction from Soil:
\text{fric}4 = W_S L_s \cos \theta_s \mu{\text{soil}}
\text{fric}_4 = W_S L_s \cos \theta_s \mu_{\text{soil}}Ls – Length of straight section between bends (ft)
θs – Angle in degrees from horizontal for straight section between bends
Drag Forces from Mud:
\text{Drag}4 = \pi D L_s \mu{\text{mud}}
\text{Drag}_4 = \pi D L_s \mu_{\text{mud}}Tension on Section:
\Delta T_4 = |\text{fric}_4| + \text{Drag}_4 – W_s L_s \sin \theta_s
\Delta T_4 = |\text{fric}_4| + \text{Drag}_4 - W_s L_s \sin \theta_sCumulative Pull Load:
T_4 = \Delta T_4 + T_3
T_4 = \Delta T_4 + T_3
Curved Section D-E
h5 = R_2 \left( 1 – \cos\left(\frac{\theta_{C2}}{2}\right) \right) \~\ I_5 = \pi (D – t)^3 \frac{t}{8} \ j_5 = \left( E\frac{ I_5}{T_{\text{avgassumed5}}} \right)^{\frac{1}{2}} \~\ U_5 = \frac{L_{\text{arc2}}}{J_5} \~\ X_5 = 3 \frac{L_{\text{arc2}}}{12} – \left( \frac{j_5}{2} \right) \tanh\left( \frac{U_5}{2} \right) \~\ Y_5 = 18 \left( \frac{L_{\text{arc2}}}{12} \right)^2 – j_5^2 \left( 1 – \frac{1}{\cosh\left(\frac{U_5}{2}\right)} \right) \~\ N_5 = \frac{T_{\text{avgassumed5}} h_5 – W_s \cos\left(\frac{\theta_{C2}}{2}\right) Y_5}{X_5}
h5 = R_2 \left( 1 - \cos\left(\frac{\theta_{C2}}{2}\right) \right) \\~\\ I_5 = \pi (D - t)^3 \frac{t}{8} \\ j_5 = \left( E\frac{ I_5}{T_{\text{avgassumed5}}} \right)^{\frac{1}{2}} \\~\\ U_5 = \frac{L_{\text{arc2}}}{J_5} \\~\\ X_5 = 3 \frac{L_{\text{arc2}}}{12} - \left( \frac{j_5}{2} \right) \tanh\left( \frac{U_5}{2} \right) \\~\\ Y_5 = 18 \left( \frac{L_{\text{arc2}}}{12} \right)^2 - j_5^2 \left( 1 - \frac{1}{\cosh\left(\frac{U_5}{2}\right)} \right) \\~\\ N_5 = \frac{T_{\text{avgassumed5}} h_5 - W_s \cos\left(\frac{\theta_{C2}}{2}\right) Y_5}{X_5}
θC2 – Angle in degrees from horizontal for curved section 2
R2 – Radius of curvature of curve section 2 (ft)
Larc2 – Length of curved section 2(ft)
Friction from Soil:
\text{fric}5=|N_5\mu{soil}|
\text{fric}_5=|N_5\mu_{soil}|Drag Forces from Mud:
Drag_5=\pi L_{arc2}\mu_{mud}
Drag_5=\pi L_{arc2}\mu_{mud}Tension on Section:
\Delta T_5 = 2|\text{fric}5| + \text{Drag}_5 – W_s L{\text{arc2}} \sin\left(\frac{\theta_{C2}}{2}\right)
\Delta T_5 = 2|\text{fric}_5| + \text{Drag}_5 - W_s L_{\text{arc2}} \sin\left(\frac{\theta_{C2}}{2}\right)
Cumulative Pull Load:
T_6=\Delta T_6+T_5
T_6=\Delta T_6+T_5
Straight Section E-F
Friction from Soil: fric_{6}=W_SL_2\cos\theta_{S2}\mu_{Soil}
\text{fric}_{6}=W_SL_2\cos\theta_{S2}\mu_{Soil}Drag Forces from Mud:
\text{Drag}6=\pi DL_2 \mu{mud}
\text{Drag}_6=\pi DL_2 \mu_{mud}Tension on Section:
\Delta T_6 = 2|\text{fric}6| + \text{Drag}_6 – W_s L_1 \sin\theta{s1}
\Delta T_6 = 2|\text{fric}_6| + \text{Drag}_6 - W_s L_1 \sin\theta_{s1}∆𝑇6 = |𝑓𝑟𝑖𝑐6| + 𝐷𝑟𝑎𝑔6 + 𝑊𝑠𝐿1𝑠𝑖𝑛𝜃𝑠12 – Length of the straight section 2
θS2 – Angle in degrees from horizontal for straight section 2
Cumulative Pull Load:
T_6=\Delta T_6+T_5
T_6=\Delta T_6+T_5
Total pull load of the pipe:
T_{total}=\Delta T_2+\Delta T_3+\Delta T_4+\Delta T_5+\Delta T_6
T_{total}=\Delta T_2+\Delta T_3+\Delta T_4+\Delta T_5+\Delta T_6Ttotal – Total pull load of the pipe (lbf)
Maximum Pull Force, F:
f_b = \frac{E \cdot D \cdot 12}{24 R_1}\~\
\text{Area} = \frac{\pi}{4} \cdot (D^2 – ID^2)\~\
SMYS_{\text{code}} = \text{codedesign}{factor} SMYS\~\ F = \left[ \left(\frac{SMYS{\text{code}} fl}{fs}\right) – f_b \right] \text{Area}\~\
\text{Result}{\text{pull}} = \begin{cases} \text{Pass}, \text{if } T{\text{total}} < F \
\text{Fail}, \text{else}
\end{cases}
f_b = \frac{E \cdot D \cdot 12}{24 R_1}\\~\\
\text{Area} = \frac{\pi}{4} \cdot (D^2 - ID^2)\\~\\
SMYS_{\text{code}} = \text{codedesign}_{factor} SMYS\\~\\
F = \left[ \left(\frac{SMYS_{\text{code}} fl}{fs}\right) - f_b \right] \text{Area}\\~\\
\text{Result}_{\text{pull}} =
\begin{cases}
\text{Pass}, \text{if } T_{\text{total}} < F \\
\text{Fail}, \text{else}
\end{cases}
ID – Internal Pipe Diameter (inch)
fs – Safety Factor
fl – Maximum Load Factor
F – Maximum pull force (lbf)
Tensile Stress at a Point:
T_{Stress}=\frac{T_{point}}{Area}
T_{Stress}=\frac{T_{point}}{Area}Allowable Tensile Stress:
T_{Stress.allow}=0.9SMYS
T_{Stress.allow}=0.9SMYSBending Stress at a point:
B_{stress}=\frac{ED12}{24R1}
B_{stress}=\frac{ED12}{24R1}Allowable Bending Stress:
a_3 = 0.75 \, \text{SMYS}\~\ b_3 = \left( 0.84 – \frac{1.74 \times \text{SMYS} \times D}{Et} \right) \text{SMYS} \~\ C3 = \left( 0.72 – \frac{0.58 \times \text{SMYS} \times D}{Et} \right) \text{SMYS} \~\ B_{\text{stress.allow}} = \begin{cases}
a3 & \text{if } \frac{D}{t} \leq \frac{1500000}{\text{SMYS}} \~\
b3 & \text{if } \frac{1500000}{\text{SMYS}} \leq \frac{D}{t} \leq \frac{3000000}{\text{SMYS}} \~\
c3 & \text{otherwise}
\end{cases}
a_3 = 0.75 \, \text{SMYS}\\~\\ b_3 = \left( 0.84 - \frac{1.74 \times \text{SMYS} \times D}{Et} \right) \text{SMYS} \\~\\ C3 = \left( 0.72 - \frac{0.58 \times \text{SMYS} \times D}{Et} \right) \text{SMYS} \\~\\ B_{\text{stress.allow}} = \begin{cases}
a3 & \text{if } \frac{D}{t} \leq \frac{1500000}{\text{SMYS}} \\~\\
b3 & \text{if } \frac{1500000}{\text{SMYS}} \leq \frac{D}{t} \leq \frac{3000000}{\text{SMYS}} \\~\\
c3 & \text{otherwise}
\end{cases}
Hoop Stress at a point:
H_{stress}=\frac{\Delta PD}{2t}
H_{stress}=\frac{\Delta PD}{2t}Allowable Hoop Stress:
a = 0.88E \left( \frac{t}{D} \right)^2 \~\ b = {0.45 \, SMYS + 0.18 \, a} \~\ c = \frac{1.31SMYS}{1.15 + \left( \frac{SMYS}{a} \right)}\~\ d = 6.2SMYS\~\ H_{stress.allow}=
a = 0.88E \left( \frac{t}{D} \right)^2 \\~\\ b = {0.45 \, SMYS + 0.18 \, a} \\~\\ c = \frac{1.31SMYS}{1.15 + \left( \frac{SMYS}{a} \right)}\\~\\ d = 6.2SMYS\\~\\ H_{stress.allow}=
- if 0.55𝑆𝑀𝑌𝑆≥𝑎
- if 0.55 SMYS ≤𝑎≤1.6 𝑆𝑀𝑌𝑆
- if 1.6 SMYS ≤𝑎≤6.2 𝑆𝑀𝑌𝑆
- otherwise
Unity Check – Tensile and Bending Stress:
TB=\frac{T_{stress}}{0.9SMYS}+\frac{B_{stress}}{B_{stress.allow}}
TB=\frac{T_{stress}}{0.9SMYS}+\frac{B_{stress}}{B_{stress.allow}}Unity Check – Tensile, Bending & Hoop Stress:
TBH = A^2 + B^2 + 2v|A|B \~\ A = {(T_{stress} + B_{stress} – 0.5H_{stress})}\frac{1.25}{SMYS} \~\ B = \frac{1.5H_{stress}}{H_{stress.allow}}
TBH = A^2 + B^2 + 2v|A|B \\~\\ A = {(T_{stress} + B_{stress} - 0.5H_{stress})}\frac{1.25}{SMYS} \\~\\ B = \frac{1.5H_{stress}}{H_{stress.allow}}
Input Parameters
Drill Path/Borehole Design*:
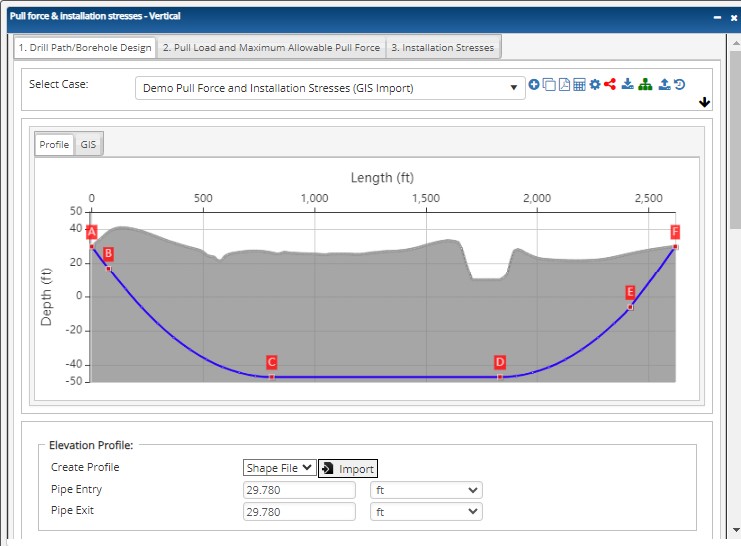
Elevation Profile
- Pipe Entry (ft)
- Pipe Exit (ft)

Downslope: Straight Section A – B
- Pipe Entry Angle A – B [degree]
- Measured Length [ft]
Downslope: Curved Section B – C
- Bend Angle B – C [degree]
- Radius of Curvature B – C [ft]
- Measured Length [ft]
Straight Section C – D
- Horizontal Angle[degree]
- Measured Length [ft]
Upslope: Curved Section D – E
- Bend Angle D – E [degree]
- Radius of Curvature D – E [ft]
- Measured Length [ft]
Upslope: Straight Section E- F
- Pipe Exit Angle E – F [degree]
- Measured Length [ft]
*The design changes based on borehole design (section configuration)
Pull Load and Maximum Allowable Pull Force:
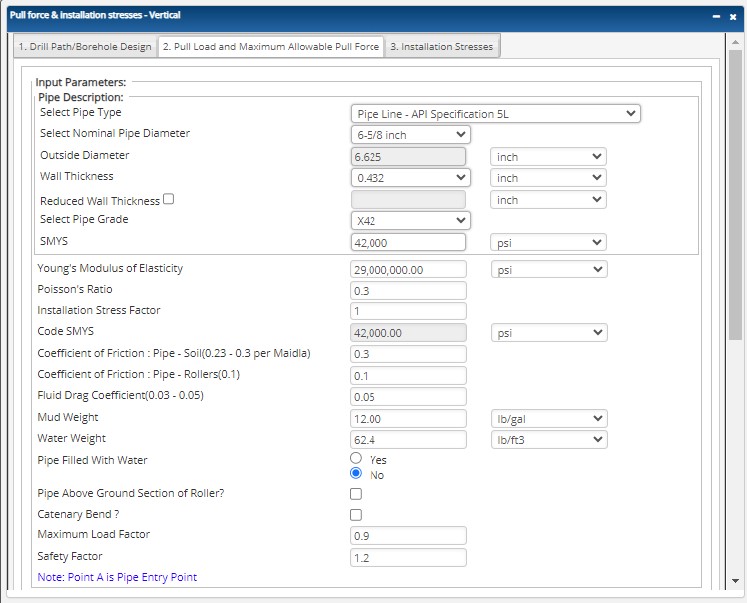
- Pipe Outside Diameter (in)
- Pipe Wall Thickness(in)
- Pipe Grade
- SMYS – Specified Minimum Yield Strength(psi)
- Poisson’s Ratio
- Young’s Modulus of Elasticity(psi)
- Code Design Factor
- Code SMYS
- Coefficient of Friction: Pipe – Soil (0.21 – 0.3)
- Coefficient of Friction: Pipe – Rollers (0.1)
- Fluid Drag Coefficient (0.03 – 0.05) (psi)
- Mud Weight (lbs./gal)
- Water Weight(lbs./ft3)
- Pipe Filled with Water:
- Pipe Above Ground Section of Roller?
- Pipe Section Above Ground Section on Rollers (ft)
- Angle of Pipe Above Ground on Rollers (°)
- Maximum Load Factor
- Safety Factor
- Note: Point A is Pipe Entry Point
Outputs/Reports
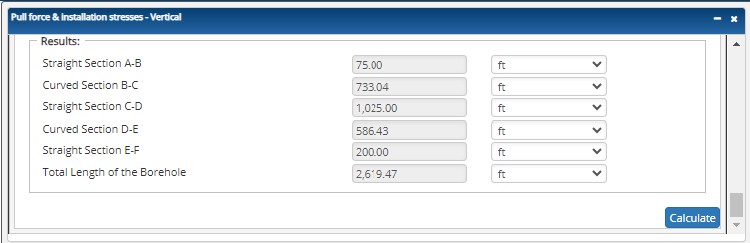
- Straight Section A-B (ft)
- Curved Section B-C (ft)
- Straight (Downslope) Section C-D (ft)
- Curved Section D-E (ft)
- Straight Section E-F (ft)
- Total Length of the Borehole (ft)
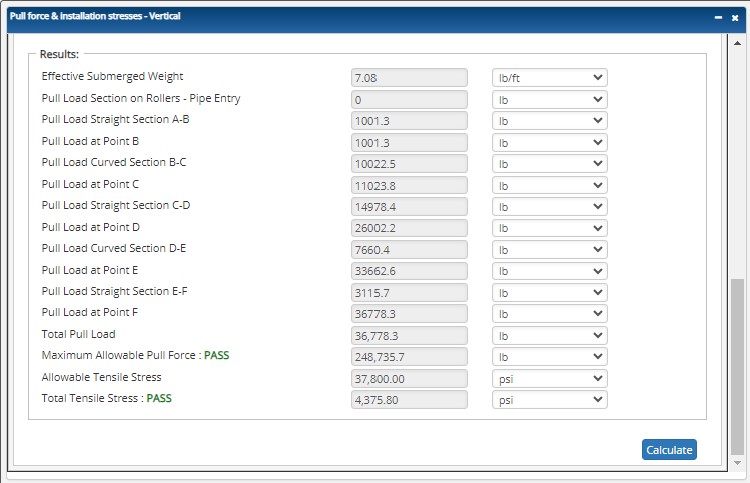
- Effective Submerged Weight (lb./ft)
- Pull Load Section on Rollers (lb)
- Pull Load Straight Section A-B (lb)
- Pull Load at Point B (lb)
- Pull Load Curved Section B-C (lb)
- Pull Load at Point C (lb)
- Pull Load Straight (Downslope) Section C-D (lb)
- Pull Load at Point D (lb)
- Pull Load Curved Section D-E (lb)
- Pull Load at Point E (lb)
- Pull Load Straight Section E-F (lb)
- Total Pull Load at Point F (lb)
- Maximum Allowable Pull Force (lb)
- Allowable Tensile Stress (psi)
- Total Tensile Stress (psi)
Pass/Fail:
Select Point of Interest (B)
- Tensile Stress(psi)
- Allowable Tensile Stress(psi)
- Bending Stress(psi)
- Allowable Bending Stress(psi)
- Hydrostatic Mud Pressure(psi)
- External Hoop Stress(psi)
- Allowable Elastic Hoop Buckling
- Combined Load Interaction at Point
- Unity Check: Tensile and Bending
- Unity Check: Tensile, Bending, and External Hoop
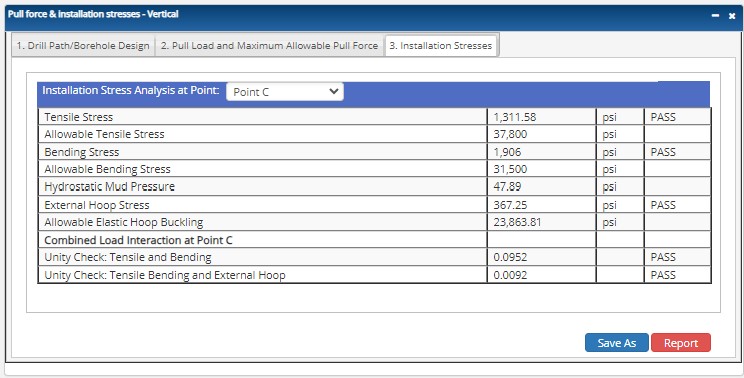
Select Point of Interest (C)
- Tensile Stress(psi)
- Allowable Tensile Stress(psi)
- Bending Stress(psi)
- Allowable Bending Stress(psi)
- Hydrostatic Mud Pressure(psi)
- External Hoop Stress(psi)
- Allowable Elastic Hoop Buckling
- Combined Load Interaction at Point
- Unity Check: Tensile and Bending
- Unity Check: Tensile, Bending, and External Hoop
Select Point of Interest (D)
- Tensile Stress(psi)
- Allowable Tensile Stress(psi)
- Bending Stress(psi)
- Allowable Bending Stress(psi)
- Hydrostatic Mud Pressure(psi)
- External Hoop Stress(psi)
- Allowable Elastic Hoop Buckling
- Combined Load Interaction at Point
- Unity Check: Tensile and Bending
- Unity Check: Tensile, Bending, and External Hoop
Select Point of Interest (E)
- Tensile Stress(psi)
- Allowable Tensile Stress(psi)
- Bending Stress(psi)
- Allowable Bending Stress(psi)
- Hydrostatic Mud Pressure(psi)
- External Hoop Stress(psi)
- Allowable Elastic Hoop Buckling
- Combined Load Interaction at Point
- Unity Check: Tensile and Bending
- Unity Check: Tensile, Bending, and External Hoop
Select Point of Interest (F)
- Tensile Stress(psi)
- Allowable Tensile Stress(psi)
- Bending Stress(psi)
- Allowable Bending Stress(psi)
- Hydrostatic Mud Pressure(psi)
- External Hoop Stress(psi)
- Allowable Elastic Hoop Buckling
- Combined Load Interaction at Point
- Unity Check: Tensile and Bending
- Unity Check: Tensile, Bending, and External Hoop
References
- Willoughby, David (2005). Horizontal Directional Drilling, McGraw-Hill, New York, ISBN 0-87814-395-5. v.
- Willoughby, David, Training – Horizontal Directional Drilling, TTI, November 2016
- HDD Consortium. (2001). Horizontal directional drilling, good practices guidelines, HDD Consortium.
- Horizontal Directional Drilling Training Manual, Horizontal Drilling International, February 1999
- Skonberg, Eric R. II. Muindi, Tennyson M. (2014). Pipeline Design for Installation by Horizontal Directional Drilling, American Society of Civil Engineers. Horizontal Directional Drilling Design Guideline Task Committee.
- “Installation of Pipelines by Horizontal Directional Drilling”, PRCI Report PR-227-9424
- Nayyar, Mohinder L. (1992). Piping Handbook, 6th Edition, McGraw-Hill, New York, NY.
- AWWA (2006), PE Pipe Design and Installation, M55, American Water Works Association, Denver, CO
- ASTM (1962), PPI Handbook
AUGER BORING – Casing is jacked into the ground as a rotating auger works simultaneously to remove the excavated soil. It is commonly used in applications where settlement is a concern: under highways, railways and levies. Also known as a dry bore.
BENTONITE – A natural clay material used as a basic ingredient for drilling muds and lubricants to facilitate ease of installation.
BORE OR BOREHOLE – drilling term – The elongated cavity created by the drilling process. Often the borehole is not a void, but rather a hole filled with drilling mud and cuttings. Well casing is pulled or pushed into the borehole to complete a well.
CASING – drilling term – The non-perforated or non-slotted pipe that comprises the entry and exit sections of a horizontal well, as opposed to the well screen. Surface casing is a pipe that is set through loose surficial deposits to stabilize the bore, so the deeper sections can be drilled without difficulty from caving or collapse in the upper section of the borehole.
CROSSING – A pipeline installation designed to pass beneath a surface obstruction. Examples of crossings include roads, railway tracks, water bodies, pipeline corridors, and utilities.
DRILLING MUD – drilling material – aqueous slurry that is used during drilling to transport drill cuttings from the borehole, prevent borehole collapse and provide lubrication for the drill string. Most horizontal drilling uses drilling mud of some sort, although in some conditions it is possible or preferable to drill using air or water. Drilling mud made be made using the mineral bentonite, synthetic or natural polymers, or some combination of the two.
DRILL RIG – A trenchless machine that installs pipes and cables by drilling a pilot hole that can be enlarged (if necessary), and then pulling the product line.
ENTRY POINT – The starting location of the crossing where the drill enters the ground.
EXIT POINT – The end location of the crossing.
FORWARD REAMER – drilling tool – A type of reamer used to enlarge the diameter of the borehole in a blind or single-entry well.
HORIZONTAL DIRECTIONAL DRILLING (HDD) – A surface-based trenchless technology that involves a horizontal bore under the surface along a planned pathway. Once the HDD creates a bore of a suitable size – which may require one or multiple passes by the drilling apparatus – the conduit or pipe is pulled into the bore and connections are made to the appropriate utilities.
OPEN CUT – Underground construction method involving excavation from ground level to the level required for the installation, maintenance, or inspection of a pipe, conduit, or cable. Upon completion of the work, the trench is backfilled, and the surface restored. Backhoe excavation is an example of open-cut construction.
PILOT BORE – drilling term – The initial boring made in a horizontal well installation. The pilot bore is steered, using any of several technologies, from a designated entry point, along a predetermined bore path, to a designated endpoint, either at the ground surface or at depth. The pilot bore subsequently may be reamed to a larger diameter to accommodate the desired size well screen and casing.
PIPE PULLING – Method used to replace small diameter pipes by attaching new product pipe to the existing pipe, which is then pulled out of the ground.
POTHOLE – drilling term – a small hole excavated from the surface to a buried utility in order to provide positive verification of its location.
REAMER – drilling tool – a cutting tool used to enlarge the diameter of a borehole after the pilot bore has been drilled.
FAQ
-
HDD Power Tool?
Horizontal Directional Drilling or HDD, is a method of installing steel or polyethylene pipe, conduit, or cables in a designed bore path, by using a drilling rig, with a minimal footprint to the environment. HDD process represents a significant improvement over traditional open cut and disturbance methods for installing pipelines beneath obstructions, such as rivers or roads, which require specialized construction attention. To take full advantage of the benefits offered by horizontal directional drilling and produce designs which can be efficiently executed in the field, design engineers need a working knowledge of the process with the appropriate HDD software. Check Out
-
HDD – Version Capability Matrix
Calculations and functions involving the capabilities that HDD can provide. Check Out
-
HDDPT Process to Import KML/KMZ and Shape Files?
Here relies the step-by-step procedure of importing files and having them project accurate calculations to your reports. Check Out
-
How to Calculate a Compound Angle?
The HDD PowerTool program will calculate the compound data based on the horizontal and vertical data you enter.
For example: In the below screenshot, under curve, select the compound. You may then input 2 data elements for the horizontal and vertical part of the bend. The program will then calculate the compound. Check Out
-
Update on the Latest Technical Capabilities of the HDDPT?
Steel cased crossings have been used to avoid load considerations, unstable soil conditions, third party mechanical damage or when conditions dictated by regulatory or sound engineering practices. However, due to a variety of factors such design, installation, construction practices, materials, coatings, etc. have caused problems with protecting the carrier pipe within the casing. Check Out
-
Combined Stresses and Limitations for Both Liquid & Gas HDD?
HDD combined stresses can be analyzed by calculating the maximum shear stress on a small element in the pipeline. Maximum shear stress should be limited to 45% of the SMYS of the pipe (ASME/ANSI B31.4). This is in accordance to PRCI report PR-227-9424 from the findings and conclusions of those companies who performed and approved the research work, However, the question comes up from time to time that the B31.4 code can be converted to B31.8’s code application. Check Out
-
Hydraulic Fracture Analysis Geological Layers Data Input?
Some typical values of soil friction angle are given below for different USCS soil types at normally consolidated condition unless otherwise stated. These values should be used only as guideline for geotechnical problems; however, specific condition of each engineering problem often needs to be considered for an appropriate choice of geotechnical parameters. Check Out
